Answer:
a) t = 14.2 s , v = -92 m / s , b) v = - 59.16 m / s , t = 0.916 s
c) t = 7.75 s , x = 387.5 m
d) t = 10.64 s , x = 463.9 m , v = 92.2 m / s
Step-by-step explanation:
This is an exercise in kinematics, suppose we take the upward direction as positive
a) is thrown up vertically.
Let's use the equation
y = y₀ + v₀ t - ½ g t²
When reaching the ground y = 0, the initial height is y₀ = 300 m and the initial velocity is v₀ = + 50m / s, to simplify we use g = 10 m /s² as the value of the acceleration of gravity, for a more exact calculation we can must use 9.80 m /s²
0 = y₀ + v₀t - ½ g t²
½ 10 t² - 50 t - 300 = 0
Let's solve the quadratic equation
t² - 10 t - 60 = 0
t = [10 ±√ (10² + 4 60)] / 2
t = [10 ± 18.4] / 2
t₁ = 14.2 s
t₂ = -4.2 s
since time must be a positive quantity, the correctors result t = 14.2 s
the speed at this point is
v = v₀ - g t
v = 50 - 10 14.2
v = -92 m / s
the sign indicates that the body is going down
b) in this case the initial velocity is vo = -50 m / s
let's calculate the velocity on the ground
v² = v₀² - 2g (y-y₀)
v² = 50 2 - 2 10 ((0- 300)
v² = 3500
v = + - 59.16 m / s
as the body is going down the correct sign is the negative
v = - 59.16 m / s
the time it takes to arrive is
v = v₀ - g t
t = (v₀ - v) / g
t = (-50 + 59.16) / 10
t = 0.916 s
c) the velocity is horizontal (vox = 50 m / s), this implies that the vertical velocity is zero voy = 0
y = y₀ + v₀ t - ½ g t²
0 = 300 + 0 - ½ 10 t²
t = √ (2 300/10)
t = 7.75 s
the horizontal displacement at this time is
x = v₀ₓ t
x = 50 7.75
x = 387.5 m
d) as it is thrown with an angle let's find each component of the velocities
v₀ₓ = v₀ cos 30
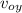 = v₀ sin 30
= v₀ sin 30
v₀ₓ = 50 cos 30 = 43.3 m / s
v_{oy} = 50 sin 30 = 25 m / s
we look for the time of descent
y = y₀ + v_{oy} t - ½ g t²
0 = y₀ + v_{oy} t - ½ g t²
0 = 300 + 25 t - ½ 10 t²
t² - 5t - 60 = 0
we solve the second degree equation
t = [5 ±√ (5² + 4 60)] / 2
t = [5 ± 16.28] / 2
t₁ = 10.64 s
t₂ = -5.64 s
since the time must be positive the result is t = 10.64 s
the range on the x axis is
x = v₀ₓ t
x = 43.6 10.64
x = 463.9 m
the ground speed is
v_{y} =
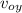 - g t
- g t
v_{y} = 25 - 10 10.64
v_{y} = -81.4 m / s
speed is
v = √ (v₀ₓ² + v_{y}²)
v = √ (43.3² + 81.4²)
v = 92.2 m / s