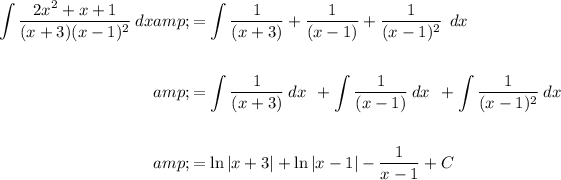Question

Please help me it is integral calculus

Answer 1

Explanation:

use the properties of integration to simplify, then take the integral.

Answer 2

First, express the fraction in partial fractions

Write it out as an identity.

As the denominator has a repeated linear factor, the power of the repeated factor tells us the number of times the factor should appear in the partial fraction. A factor that is squared in the original denominator will appear in the denominator of two of the partial fractions - once squared and once just as it is:

`(2x^2+x+1)/((x+3)(x-1)^2) \equiv (A)/((x+3))+(B)/((x-1))+(C)/((x-1)^2)`

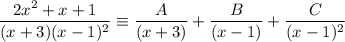
Add the partial fractions:

`(2x^2+x+1)/((x+3)(x-1)^2) \equiv (A(x-1)^2+B(x+3)(x-1)+C(x+3))/((x+3)(x-1)^2)`

Cancel the denominators from both sides of the original identity, so the numerators are equal:

`2x^2+x+1 \equiv A(x-1)^2+B(x+3)(x-1)+C(x+3)`

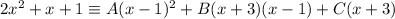
Now solve for A and C by substitution.

Substitute values of x which make one of the expressions equal zero (to eliminate all but one of A, B and C):

`\begin{aligned}x=1 \implies 2(1)^2+(1)+1 & = A(1-1)^2+B(1+3)(1-1)+C(1+3)\\4 & = 4C\\\implies C & =1\end{aligned}`

`\begin{aligned}x=-3 \implies 2(-3)^2+(-3)+1 & = A(-3-1)^2+B(-3+3)(-3-1)+C(-3+3)\\16 & = 16A\\\implies A & =1\end{aligned}`

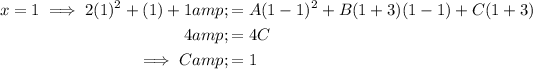
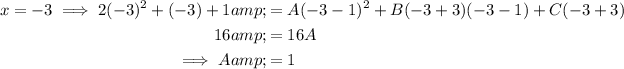
Find B by comparing coefficients:

`\begin{aligned}2x^2+x+1 & = A(x^2-2x+1)+B(x^2+2x-3)+C(x+3)\\ & = (A+B)x^2+(2B-2A+C)x+(A-3B+3C)\end{aligned}`

`\begin{aligned}\implies 2x^2 & = (A+B)x^2\\2 & = A + B\\\textsf{substituting found value of A}: \quad2 & = 1+B\\\implies B & = 1 \end{aligned}`

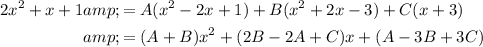
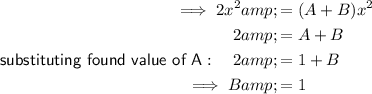
Replace the found values of A, B and C in the original identity:

`\implies (2x^2+x+1)/((x+3)(x-1)^2) \equiv (1)/((x+3))+(1)/((x-1))+(1)/((x-1)^2)`

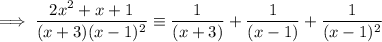
Now integrate:

`\begin{aligned}\displaystyle \int (2x^2+x+1)/((x+3)(x-1)^2)\:dx &=\int (1)/((x+3))+(1)/((x-1))+(1)/((x-1)^2)\:\:dx\\\\& =\int (1)/((x+3))\:dx\:\:+ \int(1)/((x-1))\:dx\:\:+\int (1)/((x-1)^2)\:dx\\\\& = \ln |x+3|+ \ln |x-1|-(1)/(x-1)+C\end{aligned}`