Question

Find the equation that passes through points A and B

Answer 1

Explanation:

A(1,7); B(-3,-1); slope m =(-1-7)/-3-1) = -8/-4 = 2

Equation of a line AB is

((y-y1) = m(x-x1)

y - 7 = 2(x-1)

y - 7 = 2x-2

y = 2x + 5

Answer 2

y = 2x +5

Explanation:

Equation of a line

The point-slope form of the equation of a line is:

y - k = m ( x - h )

Where m is the slope and (h,k) is a point through which the line passes.

Suppose we know the line passes through points A(x1,y1) and B(x2,y2). The slope can be calculated with the equation:

`\displaystyle m=(y_2-y_1)/(x_2-x_1)`

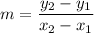
The image provides two points A(1,7) and B(-3,-1), thus the slope is:

`\displaystyle m=(-1-7)/(-3-1)`

`\displaystyle m=(-8)/(-4)`

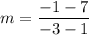
m = 2

Now we apply the point-slope form taking the point (1,7):

y - 7 = 2 ( x - 1 )

Operating:

y - 7 = 2x - 2

Adding 7:

y = 2x +5