Question

consider f(x) = x^3 + x^1 would the function be even, odd, neither,or not enough information to determine

Answer 1

The function is odd

Explanation:

Even functions and odd functions are those which satisfy particular symmetry relations as follows:

f(x) is even if f(-x) = f(x)

f(x) is odd if f(-x) = -f(x)

Not all functions are eligible for being even or odd.

Considering

`f(x)=x^3+x^1`

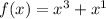
Let's find f(-x)

`f(-x)=(-x)^3+(-x)^1`

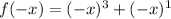
Since
`(-x)^3=(-x)(-x)(-x)=-x^3`

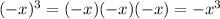
And
`(-x)^1=-x:`

`f(-x)=-(x)^3-(x)^1`

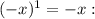
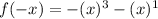
Factoring by -1:

`f(-x)=-((x)^3+(x)^1)`

The expression in parentheses if f(x), thus:

`f(-x)=-f(x)`

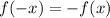
And the function is odd.