Answer:
The base is 24 cm long.
Explanation:
Equations
Let's call
b= base of the triangle
h=height of the triangle
The base is 9 cm longer than the height, thus:
b = h + 9
The area of the triangle is:

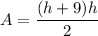
And its value is 180:
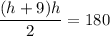
Multiplying by 2:

Operating:
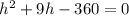
Factoring:

The only valid positive solution is:
h = 15 cm
And b = h + 9 = 24
b = 24 cm
The base is 24 cm long.