Question

A carpenter is building a rectangular shed with a fixed perimeter of 68 ft. What are the dimensions of the largest shed that can be built? What is its area?

The dimensions of the largest shed are _____ by _____.
The area of the largest shed is _____.

Answer 1

The dimensions of the largest shed are 17 ft by 17 ft.

The area of the largest shed is 289 sq ft.

Explanation:

Given that:

Fixed perimeter of the rectangular shed = 68 ft

To find:

The dimensions of the largest shed that can be built = ?

And

Area of the largest shed that can be built = ?

Solution:

First of all, let us have a look at the formula for perimeter of a rectangular shape shed:

Perimeter of a rectangle = 2
`*` (Length + Width)

68 = 2
`*` (Length + Width)

Length + Width = 34

The largest possible values are only in the case when the rectangle is a square.

i.e. Length = Width

Therefore values of length and width are:

Length = Width =
`(34)/(2) = 17\ ft`

Area of a square is given by the formula:

`A = Side * Side`

`A` = 17
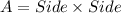
`*` 17 = 289 sq ft

The answer is:

The dimensions of the largest shed are 17 ft by 17 ft.

The area of the largest shed is 289 sq ft.