Answer:
The position of the object at
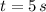 is 130.167 meters.
is 130.167 meters.
Step-by-step explanation:
Let
![a(t) = 5\cdot t\,\left[(m)/(s^(2)) \right]](https://img.qammunity.org/2021/formulas/physics/college/k4emt6u8ct8yq3pce6y4gw6f2qy1n0jv1n.png) the acceleration experimented by the object along the x-axis. We obtain the equation for the position of the object by integrating in acceleration formula twice:
the acceleration experimented by the object along the x-axis. We obtain the equation for the position of the object by integrating in acceleration formula twice:
Velocity
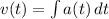 (1)
(1)
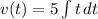
 (2)
(2)
Where
 is the initial velocity of the object, measured in meters per second.
is the initial velocity of the object, measured in meters per second.
Position
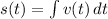 (3)
(3)
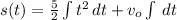
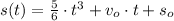 (4)
(4)
Where
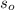 is the initial position of the object, measured in meters per second.
is the initial position of the object, measured in meters per second.
If we know that
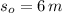 ,
,
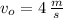 and
and
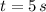 , then the position of the object is:
, then the position of the object is:

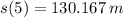
The position of the object at
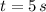 is 130.167 meters.
is 130.167 meters.