Question

A block is attached to one end of a spring so that it can bounce back and forth on a horizontal frictionless surface. The block is pulled a distance of 0.150 m away from equilibrium and released. When the block is 9.00 cm away from equilibrium, it is traveling with a speed of 0.800 m/s. If the spring constant of the spring is 60.0 N/m, what is the mass of the block?

Answer 1

m = 1.35 kg

Step-by-step explanation:

• In absence of friction, total mechanical energy must be conserved, so the sum of the initial elastic potential energy plus the initial kinetic energy, must be equal to the sum of the final kinetic energy plus the final elastic potential energy, as follows:

`U_(i) + K_(i) = U_(f) + K_(f) (1)`

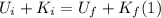
• As the block starts from rest, this means that Ki =0.
• We can express the elastic potential energy at any point , as follows:

`U = (1)/(2) * k * \Delta x^(2) (2)`

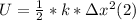
where k is the spring constant = 60.0 N/m, and Δx is the distance from

the equilibrium position.

• Replacing Ui, Uf and Kf in (1), we have:

`(1)/(2) * k *\Delta x_(i) ^(2) =(1)/(2) * k *\Delta x_(f) ^(2) +(1)/(2) * m *v ^(2) (3)`

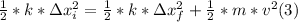
• Replacing by the givens, and solving for m, we get:

m =1.35 kg