Answer:
2.29 inches/hour
Explanation:
Given that the candle burns at a constant rate.
After time 2.7 hours, candle was 14.3 inches tall.
After time 7.1 hours, candle was 4.2 inches tall.
To find:
The constant rate at which the candle burn.
Solution:
Let the length be represented by
 and time be represented by
and time be represented by
 .
.
Let the constant rate be
 .
.
and the initial length be

The equation can be written as:
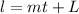
At
 = 2.7 hours ,
= 2.7 hours ,
 = 14.3 inches
= 14.3 inches
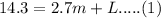
At
 = 7.1 hours ,
= 7.1 hours ,
 = 4.2 inches
= 4.2 inches
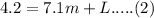
Equation (1) minus (2):
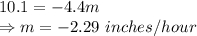
The candle burns at the rate of 2.29 inches/hour.