Answer:
18 hours = £270
Explanation:
Find how much he charges per hour. Divide:
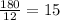
So ha charges about 15 an hour. Multiply this price by the number of hours:
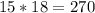
Therefore, he will charge £270 for a job that takes 18 hours.
OR
Set a proportional relationship since he charges a fixed amount:
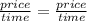
Insert the appropriate corresponding values:
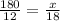
Solve for the price after 18 hours of work, represented by x. Cross multiply:
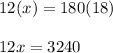
Solve for x. Use inverse operations to isolate the variable. Divide both sides by 12:
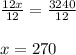
Therefore, after 18 hours, he will charge £270.
:Done