Question

the base of a triangle is 7 less than twice its height. If the area of the triangle is 102m^2, find the length of the base.

Answer 1

The length of the base will be: 17 m

Explanation:

Given the area of the triangle

`A=102\:m^2`

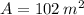
Using the formula of Area of a triangle:

`A=(1)/(2)\left(b* h\right)`

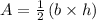
Here,

• 'b' is the base

• 'h' is the height

As the base of a triangle is 7 less than twice its height.

so

`b=2h-7`

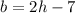
so the formula of Area of a triangle becomes

`102=(1)/(2)\left(\left(2h-7\right)* \:h\right)`

`204=h\left(2h-7\right)`

`2h^2-7h=204`

`2h^2-7h-204=0`

`\left(2h+17\right)\left(h-12\right)=0`

`2h+17=0\quad \mathrm{or}\quad \:h-12=0`

`h=-(17)/(2),\:h=12`

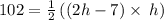
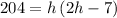
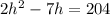
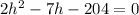
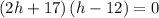
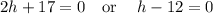
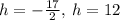
As height can not be negative, so:

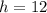`h=12` m

Hence, the length of the base:

`b=2h-7`

`= 2(12)-7`

`=24-7`

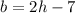
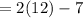
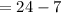
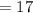`= 17` m

Therefore, the length of the base will be: 17 m