Answer:
AE:
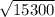
DE:150
PABCDE: 720
Explanation:
We can find AE by the pythagorean theorem,

AE is the hypotenuse so AE=c, and the leg of the triangle would be 150-30=120
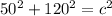
c^2=2500+14400
AE=130
We can use the pythagorean theorem again to find DE.
The base would equal 140-50=90 and the height would equal 120
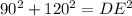
DE^2=22500
DE=150
The perimeter would be 150+140+150+130+150=720
Let me know if somethings wrong