Question

What conjecture can you make about the sum of firs 52 positive even numbers​

Answer 1

See Explanation

Explanation:

The question is incomplete. However, the available details is enough to work out a solution.

Considering the first even number: 2

In this case, n = 1

The sum of the first even term is 2.

Represent the sum as a product:

`2 = 1 * 2`

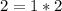
Considering the first two even numbers: 2 and 4

In this case, n = 2

The sum of the first two even terms is 6

Represent the sum as a product:

`6 = 2 * 3`

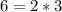
Considering the first three even numbers: 2, 4 and 6

In this case, n = 3

The sum of the first two even terms is 12

Represent the sum as a product:

`12 = 3 * 4`

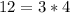
Notice the pattern from n = 1 to 3

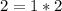`2 = 1 * 2` --- n = 1

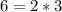`6 = 2 * 3` --- n = 2

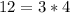`12 = 3 * 4` --- n = 3

------------------------------------

It follows that, the sum when represented as a product is:
`n * (n + 1)`

So, the sum of the first 52 even terms would be:

`52 * (52 + 1)`

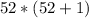
Where n = 52

`52 * (52 + 1) = 52 * 53`

`52 * (52 + 1) = 2756`

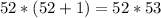
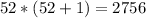
Hence, the sum of the first 52 even terms is 52 * 53 or 2756