Check the picture below.
so the height of the pole is "p", and the angle of depression to the foot of the pole is really "44 + a".
so let's use the 44° angle first

now, let's use the red triangle, namely the angle "44 + a"
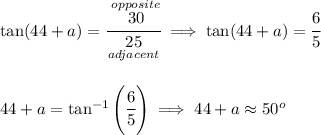
Make sure your calculator is in Degree mode.