well, we know its slope and we also know a point on it, so hmmm without further ado, we can get its equation, once we have the equation, we can get any point we like on it pretty much, so
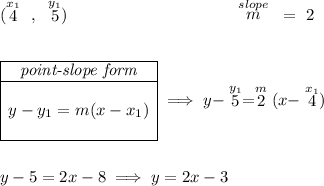
well then hmmm to get some other point hmmm let's pick a random "x" hmmm say dunno x = -13, so
![\underline{x=-13}\hspace{5em}y=2(\stackrel{x}{-13})-3\implies y=-26-3\implies \underline{y=-29} \\\\[-0.35em] ~\dotfill\\\\ ~\hfill \underset{another~point}{(-13~~,~~-29)}~\hfill](https://img.qammunity.org/2023/formulas/mathematics/college/2cr0pcbwrgm1kajxxepn26dstmcwczclgz.png)