Question

If a football is thrown upward, its height above the

ground is given by the equation s=-16t^2+Vot+so, where

Vo and so are the initial velocity and intial height of the

Football, respectively, and t is the time in seconds.suppose

the football is thrown from the top of a building that is

192 feet tall, with an initiae speed of 96 feet per second.

a)write the polynomial that gives the height of the Football in terms

of the variable t (time)

b) what is the height of the Football after 2 seconds have

Clapsed? Will the football hit the ground after 2 seconds?

Answer 1

a) s = -16
`t^(2)` + 192t + 96

b) Height of the football after 2 seconds is 448 feet.

ii. The football would not hit the ground after 2 seconds.

Explanation:

Given:

s = -16
`t^(2)` +
`V_(o)`t +
`s_(o)`

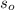
where: s is the height of the football above the ground, t is the time,
`V_(o)` is the initial velocity and
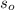`s_(o)` is the initial height.

The height of the building =
`V_(o)` = 192 feet and the initial speed of the ball =
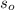`s_(o)` = 96 feet per second, then;

s = -16
`t^(2)` + 192t + 96

a) The polynomial is given as:

s = -16
`t^(2)` + 192t + 96

b) The height of the football when t = 2 seconds is;

s = -16(2) + 192(2) + 96

= -32 + 384 + 96

= -32 + 480

s = 448

The height of the football when t = 2 seconds is 448 feet.

ii. The football would not hit the ground after 2 seconds.