Answer:
a
The null hypothesis is
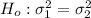
The alternative hypothesis is
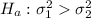
b
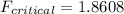
c

d
The decision rule is
Reject the null hypothesis
e
There is sufficient evidence to support the researchers claim
Explanation:
From the question we are told that
The first sample size is
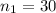
The sample variance for elementary school is

The second sample size is

The sample variance for the secondary school is
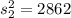
The significance level is

The null hypothesis is
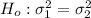
The alternative hypothesis is
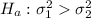
Generally from the F statistics table the critical value of
 at first and second degree of freedom
at first and second degree of freedom
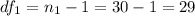 and
and
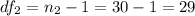 is
is
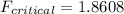
Generally the test statistics is mathematically represented as
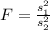
=>
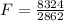
=>

Generally from the value obtained we see that
 Hence
Hence
The decision rule is
Reject the null hypothesis
The conclusion is
There is sufficient evidence to support the researchers claim