Question

If the terminal side of angle A passes through (-5,2) find the value of sec A​

Answer 1

The value of sec A = - √29/5

Explanation:

As the terminal side of angle 'A' passes through (-5,2).

so the angle is in the 2nd quadrant.

We can find the length of the hypotenuse using the Pythagorean Theorem

`c^2\:\:=\:\left(-5\right)^2\:+\:2^2`

`c^2=5^2+2^2`

`c^2=25+4`

`c^2=29`

`c=√(29)`

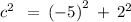
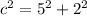
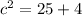
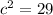
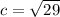
As we know that

cos A = adjacent/hypotenuse

sec A = 1/cosA

= hypotenuse/adjacent

= √29/-5

= - √29/5

Therefore, the value of sec A = - √29/5