Question

Find all the missing elements. NEED HELP PLZZZZ

Answer 1

• B=42°

• C=98°

• c=10.7 units

Explanation:

Given

• Angle A = Ф = 40°
• a=8
• b=7

We know that trigonometric ratio as

• sin Ф = opposite/hypotenuse

• cos Ф = adjacent/hypotenuse

• tan Ф = opposite/adjacent

as

sin A = sin 40 = opposite/hypotenuse

From the diagram, it is clear that the opposite of angle A is a=8 and the hypotenuse is 'c'.

also the angle

sin A = sin 40 = opposite/hypotenuse = a/c = 8/c

sin 40 = 8/c

c = 8/sin 40

c = 8/0.745

c = 10.7

so the value of c will be: 10.7 units

so

tan B = opposite/adjacent

= b/a

= 0.9

`B=\tan ^(-1)\left(0.9\right)`

`=42^(\circ \:)`

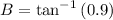
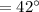
Therefore, the value of B =
`42^(\circ \:)`

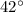
As the sum of the angles of triangle ABC is 180.

i.e.

A+B+C = 180

(40)° + (42)° + C = 180°

C = 180° - 40° - 42°

= 98°

Therefore, the value of C = 98°

Hence,

• B=42°

• C=98°

• c=10.7 units