Question

Determine whether f(x) is continuous at x = 1. if discontinuous, identify the type of discontinuity as infinite,

jump, or removable.
ax)={x^2 + 1 if x < 1
{-x^3+2 if x>1
select all that apply.
a the function is discontinuous at x =1.
b. the function is continuous at x =1.
c. infinite discontinuity
d. jump discontinuity
e removable discontinuity

Answer 1

It looks like the function is defined by

`f(x) = \begin{cases} x^2 + 1 & \text{if } x < 1 \\ -x^3 + 2 & \text{if } x > 1 \end{cases}`

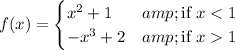
which we immediately is discontinuous at x = 1 because f(1) is not defined. One of the strict inequalities should probably have ≥ or ≤ involved.

In order for f(x) to be continuous at x = 1, we need have the one-sided limits agree:

`\displaystyle \lim_(x\to1^-) f(x) = \lim_(x\to1) (x^2+1) = 2`

`\displaystyle \lim_(x\to1^+) f(x) = \lim_(x\to1) (-x^3+2) = 1`

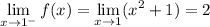
They do not agree, so f(x) is indeed discontinuous at x = 1, regardless of what value we pick for f(1). Graphically this corresponds to a jump discontinuity, where f(x) jumps from 2 down to 1 as x varies past x = 1.

[A and D]