Question

A prism with a base area of 3 m2 and a height of 4 m is dilated by a factor of 3/2

What is the volume of the dilated prism?
Enter your answer, as a
decimal, in the box.
х

Answer 1

The value of the new volume 'x' would be equal to 40.5 cm³.

Explanation:

Given

• base area = 3 m²

• height = 4m

• Dilation by a factor scale of 3/2.

We can determine the volume of the original prism by multiplying the area of the base by the height,

volume = (3 cm²)(4 cm) = 12 cm³

Dilation by a factor of 3/2 means that the dimensions of the new prism is 3/2 times that of the original object.

let 'x' be the volume of the new solid,

`(x)/(12)=\left((3)/(2)\right)^3`

`(x)/(12)=(27)/(8)`

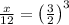
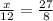
Multiply both sides by 12

`(12x)/(12)=(27\cdot \:12)/(8)`

`x=(81)/(2)`

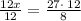
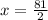
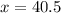`x=40.5` cm³

Therefore, the value of the new volume 'x' would be equal to 40.5 cm³.