Question

The English Channel is 35 km wide at its narrowest point, which is where most swimmers attempt to cross north to south from England to France. The current across this portion of the English Channel runs at about 2 km/h east to west.

a. If a swimmer has a relative velocity to the shore of 3 km/h north to south, how long will it take her to cross the 35 km distance?

b. What must be her velocity relative to the water if she has a velocity of 3 km/h north to south relative to the shore?

Answer 1

a.) 20.4 h

b.) 3.46 km/h

Step-by-step explanation:

Given that an English Channel is 35 km wide at its narrowest point, which is where most swimmers attempt to cross north to south from England to France. The current across this portion of the English Channel runs at about 2 km/h east to west.

a.) If a swimmer has a relative velocity to the shore of 3 km/h north to south, The resultant velocity (Vr) will be achieved by using Pythagoras theorem

Vr =
`\sqrt{2^(2) + 3^(2) }`

Vr =
`√(4 + 9)`

Vr =
`√(12)`

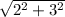
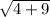
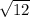
Vr = 3.46 m/s

To get how long it take her to cross the 35 km distance, Let us first find acceleration by using third equation of motion.

`V^(2) = U^(2) + 2aS`

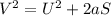
Where U = 0

Substitute all the parameters into the equation

`3.46^(2) = 2 x a x 35`

11.97 = 70a

a = 11.97/70

a = 0.17 km/h^2

The time can be found by using equation

V = U + at

let U = 0

3.46 = 0.17t

t = 3.46/0.17

t = 20.35h

b. What must be her velocity relative to the water if she has a velocity of 3 km/h north to south relative to the shore?

her velocity relative to the water will be

Vr =
`\sqrt{2^(2) + 3^(2) }`

Vr =
`√(4 + 9)`

Vr =
`√(12)`

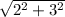
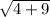
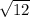
Vr = 3.46 m/s