Question

Jack has a saving account of value $65,550 at the start. The saving account has an interest rate of 3% that is compounded every six months. How long will it take Jack to have $100,000 in his account?

Answer 1

Given:

Principal value = $65,550

Rate of interest = 3% compounded every six month

To find:

The taken to have $100,000 in Jack's account.

Solution:

The formula for amount is

`A=P(1+(r)/(n))^(nt)`

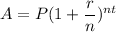
where, P is principal, r is rate of interest, n is number of times interest compounded in an year, t is time in number of years.

Interest compounded every six month. It means, interest compounded 2 times in an year.

Substitute A=100000, r=0.03 and n=2 in the above formula.

`100000=65550(1+(0.03)/(2))^(2t)`

`(100000)/(65550)=(1+0.015)^(2t)`

`1.525553=(1.015)^(2t)`

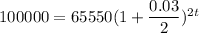
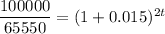
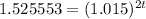
Taking log on both sides.

`\log (1.525553)=\log (1.015)^(2t)`

`\log (1.525553)=2t\log (1.015)`

`(\log (1.525553))/(2\log (1.015))=t`

`t=14.1838928`

`t\approx 14.18`

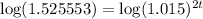
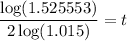
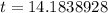
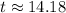
Therefore, after 14.18 year the amount will reach at $100,000 or we can say that in 15th year the amount will reach at $100,000.