Answer:
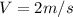
Step-by-step explanation:
Given:
Represent
- Susan's velocity with
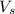
- Michael's velocity with
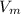
 eastwards
eastwards
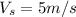 westwards
westwards
Required
Determine how fast is
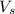 relative to
relative to
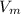
From the question, one train moves eastwards while the other moves westwards.
This means that travel in opposite travel and the relative speed (V) is calculated as:

Substitute values for
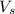 and
and
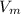
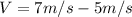
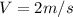
Hence, the Susan's train is moving 2m/s in relative to Michael's train