Question

Which statements verify that the solution set to lx + 31 < 5 is -8

Substituting a value into the inequality from the solution set, such as -2, will create a true statement.

* Substituting a value into the inequality from the solution set, such as 1, will create a false statement.

* Substituting a value into the inequality not from the solution set, such as 4, will create a true statement.

Substituting a value into the inequality not from the solution set, such as 6, will create a false statement.

* Substituting any value into the inequality will create a true statement.

Answer 1

Given:

Consider the inequality is
`|x+3|<5` and its solution set is
`-8<x<2`.

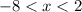To find:

The statement that verify the solution set.

Solution:

Any value into the inequality form the solution set
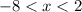`-8<x<2`, will create a true statement and any value into the inequality not form the solution set
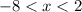`-8<x<2`, will create a false statement.

To verify the solution set set, we need to put any value form solution set into the given inequality.

Substituting a value into the inequality from the solution set, such as -2, will create a true statement.

Therefore, the correct option is A.