Question

You operate a gaming website, www.mudbeast.net, where users must pay a small fee to log on. When you charged $4 the demand was 520 log-ons per month. When you lowered the price to $3.50, the demand increased to 780 log-ons per month.

(a) Construct a linear demand function for your website and hence obtain the monthly revenue R as a function of the log-on fee x.

R(x) =

(b) Your Internet provider charges you a monthly fee of $30 to maintain your site. Express your monthly profit Pas a function of the log-on fee x.

P(x) =

Determine the log-on fee you should charge to obtain the largest possible monthly profit.

x = $

What is the largest possible monthly profit?

$

Answer 1

a)
`R(x)=-520x^2+2600x`

b)
`P(x)=-520x^2+2600x-30`

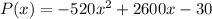
c)x=2.5

d)P(x)=3220

Explanation:

Let Demand function be D=Ax+B

We are given that When you charged $4 the demand was 520 log-ons per month

So, D = 520 , x = 4

So, 520=4A+B ----1

We are also given that When you lowered the price to $3.50, the demand increased to 780 log-ons per month.

So, D=780 , x = 3.50

So, 780=3.5A+B ----2

Substract 2 form 1

4A+B-3.5A-B=520-780

0.5A=-260

`A=(-260)/(0.5)`

A=-520

Substitute value of A in 2

780=3.5(-520)+B

780-3.5(-520)=B

2600=B

So, D=-520x+2600

Revenue = Demand (x)=(-520x+2600)(x)

a)
`R(x)=-520x^2+2600x`

b) Profit = Revenue - cost

`P(x)=-520x^2+2600x-30`

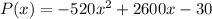
c)to obtain the largest possible monthly profit.

Equate the first derivative of profit to 0

P'(x)=-1040x+2600=0

`x=(2600)/(1040)`

x=2.5

d)Now to find the largest possible monthly profit

Substitute x = 2.5 in Profit function

`P(x)=-520(2.5)^2+2600(2.5)-30`

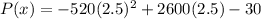
P(x)=3220