Answer:
The option D represents an interval with a positive average rate of change.
Explanation:
Let
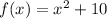 , the average rate of change on the interval
, the average rate of change on the interval
![[a,b]](https://img.qammunity.org/2021/formulas/mathematics/middle-school/dyyh30s5r4ox0wg76shisxuulr9zptlacg.png) is represented by the definition of the secant line:
is represented by the definition of the secant line:
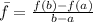 (1)
(1)
Where:
 ,
,
 - Lower and upper bounds, dimensionless.
- Lower and upper bounds, dimensionless.
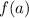 ,
,
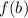 - Function evaluated at lower and upper bounds, dimensionless.
- Function evaluated at lower and upper bounds, dimensionless.
Now we proceed to check each option:
A.
 ,
,
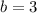
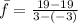
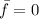
B.
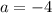 ,
,
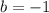
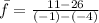
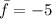
C.
 ,
,
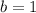
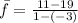
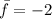
D.
 .
.
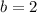
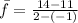
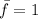
The option D represents an interval with a positive average rate of change.