Answer:
Option (2)
Explanation:
From the picture attached,
Let the rectangular coordinates (x, y) is represented by the polar coordinates (r, θ).
By applying Pythagoras theorem in ΔPAO,
PO² = AO² + AP²
r² = x² + y²
r =
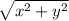
By applying tangent rule in ΔAPO,
tanθ =
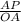
tanθ =
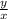
θ =
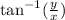
Therefore, Option 2 will be the correct option.