Question

find the value of K so that the line through the points (4,-3) and(k,7) is parallel to the line with the equation 4x+6y=10

Answer 1

-8

Explanation:

Convert the equation from standard form into slope-intercept form by....

• Moving the variable to the right-hand side and change its sign: 6y = 10 - 4x
• Divide both sides of the equation by 6 : y=
  `(5)/(3)`-
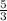  `(2)/(3)`x

• Use the commutavie property to reoder the terms : y= -
  `(2)/(3)`x +
  `(5)/(3)`

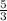
(Parallel lines have the same slope.. so the slope = -
`(2)/(3)`)

Create and equation using the slope and the point (k,7)

• Make the equation : 7 = -
  `(2)/(3)`(k) +
  `(5)/(3)`

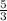
• Multiply both sides of the equation by 3 : 21 = -2k + 5

• Move the varable to the left-hand side and change its sign: 2k + 21 = 5

• Move the constant to the right-hand side and change its sign: 2k + 5 - 21

• Calculate the difference: 2k = -16

• Divide both sides of the equation by 2 : k= -8

Solution : k = -8