Answer:
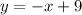
Explanation:
This is asking you to write the equation in slope-intercept form:

where:
- m is the slope
- b is the y-intercept
- x and y are any corresponding coordinate points (x,y)
You need to find the slope of the two given points using:
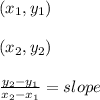
Insert the values:
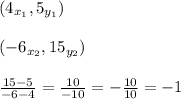
The slope of the given line is -1. Insert this value into the equation:
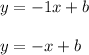
Now insert one of the given coordinate points as x and y to solve for b:

The y-intercept is 9. Insert the value:
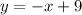
:Done