Answer:
The volume of the geometric solid produced is 391 cubic cm ⇒ A
Explanation:
When a right triangle is rotated about its vertical leg 360°, then it formed a cone its radius is the horizontal leg of the triangle and its height is the vertical lege of the triangle.
The rule of the volume of the cone is V =
 π r² h, where
π r² h, where
- r is the radius of its base
- h is the length of its height
∵ Triangle XYZ is rotated 360° about the vertical side YZ
∴ It formed a cone with a radius = XZ and a height = YZ
∵

∵ YX = 6√3
∴
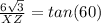
∵ tan(60) = √3
∴
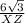 = √3
= √3
→ By using cross multiplication
∴ 6√3 = XZ(√3)
→ Divide both sides by √3
∴ 6 = XZ
∵ XZ = r and YZ = h
∴ r = 6 and h = 6√3
→ By using the rule of the cone above
∵ V =
 (π) (6)² (6√3)
(π) (6)² (6√3)
∴ V ≅ 391 cm³
∴ The volume of the geometric solid produced is 391 cubic cm