Question

The length of a rectangle is 5 more than twice the width. if the perimeter of the rectangle is 76 meters, find the dimensions of the rectangle and choose the equation that represents the perimeter:

1. 76=2w+5
2. 76=2w+2(2w+5)
3. 76=w+2w+5
4. 76=2w+(2w+5)

Answer 1

Dimensions: 11 meters by 27 meters

2.
`76=2w+2(2w+5)`

Explanation:

Finding Dimensions:

The perimeter of a rectangle can be written as
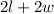`2l+2w` where
`l` is the length and
`w` is the width. It is given that the length is 5 more than twice the length of width and the perimeter of the rectangle is 76 meters.

With this information we can write:

Length =
`2w+5`

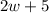
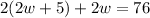`2(2w+5)+2w = 76` (perimeter of the rectangle)

`4w+10+2w=76`

`6w=66`

`w=11`

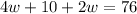
Length =
`2(11)+5`

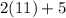
Length =
`27`

∴ The dimensions of the rectangle is 11 meters by 27 meters.

Perimeter

Since the length can be written as
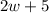`2w+5`, the equation that represents the perimeter of the rectangle is
`76=2w+2(2w+5)`