Answer:
y=-2x+19
Explanation:
For lines like these, the slope is always defined as "the change in y over the change in x" or, in equation form: m=
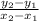 .
.
Now we have,
m= 3-7/8-6
m=-2. Since m=-2, the equation is y=-2x+b.
To find b, think about what your (x,y) points mean:
(6,7). When x of the line is 6, y of the line must be 7.
(8,3). When x of the line is 8, y of the line must be 3.
Substitute them to the equation y=-2x+b.
You can use either (x,y) point you want..the answer will be the same:
(6,7). y=mx+b or 7=-2 × 6+b, or solving for b: b=7-(-2)(6). b=19.
(8,3). y=mx+b or 3=-2 × 8+b, or solving for b: b=3-(-2)(8). b=19.
So, the equation of the line that passes through the points
(6,7) and (8,3) is y=-2x+19.