Question

Find the value of c that completes the square

x^2-x+c

Please help me with how to do this, and show me. Thank you.

Answer 1

The value of c is 1/2

Explanation:

We have to find the value of c that completes the square
`x^2-x+c`

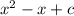
So, completing square is of form:
`a^2-2ab+b^2=(a-b)^2`

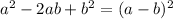
In the question given the value c can be found by breaking the middle term. we are given -x while the general formula is 2ab for middle term so, our c = 1/2 i.e 2(x)(1/2) we get x so, solving:

`x^2-x+c\\=x^2-2(x)((1)/(2))+((1)/(2))^2-((1)/(2))^2\\=(x-(1)/(2))^2-((1)/(2))^2`

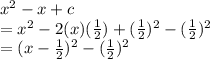
The value of c is 1/2