Question

A parabola can be drawn given a focus of

(8,-1) and a directrix of y = 3. What can be
said about the parabola?

Answer 1

If you sketch the directrix and the focus, the vertex is halfway between them. It would be (-8,-1)

The p value is the distance from the focus to the vertex and the distance between the vertex and the directrix (they are the same) p=3.

The direction of the focus from the vertex will show which way it opens. The one opens down.

Explanation:

hehe

Answer 2

• vertex: (8, 1)
• p-value: -2
• opens downward

Explanation:

The vertex of a parabola is halfway between the focus and the directrix. The p-value of the equation is half the distance from the focus to the directrix. The parabola opens around the focus, away from the directrix.

__

vertex

That is, the vertex will lie on the same vertical line, but have a y-coordinate that is the average of the y-values of the focus and directrix. Here, that means the y-value of the vertex is ...

(directrix +focus)/2 = (3 +(-2))/2 = 1

The focus is on the vertical line x=8. The coordinates of the vertex are (8, 1).

__

p-value

The parameter "p" is part of the leading coefficient of the quadratic defining the parabola. It fits into the vertex-form equation as shown here:

`y=\frac{1}{4\mathbf{p}}(x-h)^2+k\qquad\text{vertex at $(h,k)$}`

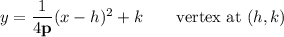
The value of p is the distance from the focus to the vertex. When the focus is below the vertex, as here, the value of p will be negative.

p = (focus y-value) - (vertex y-value) = (-1) -1

p = -2

__

opening

The focus lies "inside" the parabola, and the directrix lies "outside." The parabola "wraps around" the focus, away from the directrix. When the focus is below the directrix, the parabola opens downward. (The leading coefficient of the equation is negative, telling you the same thing.

_____

Additional comment

The parabola is the set of points equidistant from the focus and directrix. Thus it should come as no surprise that the vertex is equidistant from the focus and directrix. They lie on the same vertical line when the directrix is horizontal.

The latus rectum is the line segment parallel to the directrix, through the focus, with end points on the parabola. Its end points always lie on a line with slope ±1/2 through the vertex. This can help you find (or verify) the focus graphically.