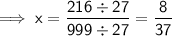Answer:
Let x equal the recurring decimal:
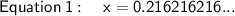
Create another number with recurring 216s by multiplying the above expression by 1000:
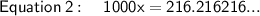
Subtract Equation 1 from Equation 2 to eliminate the recurring digits after the decimal:
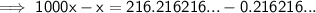

Divide both sides by 999:

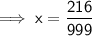
Simplify: