Question

Identify the solution and graph of the given inequality.
34 > 3(2 − x)

Answer 1

Solving the inequality we get x>-9.33

The graph is shown in figure attached.

Explanation:

We need to solve the inequality:
`34 > 3(2-x)`

Solving:

`34 > 3(2-x)\\`

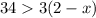
Switching the sides , reversing the inequality and Multiply 3 with terms inside the bracket

`3(2-x)<34\\6-3x<34`

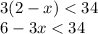
Subtract 6 from both sides

`6-3x-6<34-6\\-3x<28`

Divide both sides by -3 and inequality will be reversed

`(-3x)/(-3) >(-28)/(3) \\x>(-28)/(3)\\x>-9.33`

Solving the inequality we get x>-9.33

The graph is shown in figure attached.