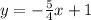Question

Write, in slope intercept form, the equation of the line that passes through (8, -9) and has a slope of -5/4.

Answer 1

The equation in the slope-intercept form will be:

`y=-(5)/(4)x+1`

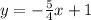
Explanation:

Given

• slope = m = -5/4

• point = (8, -9)

As we know that the equation of a line in point-slope form is

`y-y_1=m\left(x-x_1\right)`

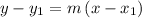
substituting the values m = -5/4 and point = (8, -9)

`y-\left(-9\right)=(-5)/(4)\left(x-8\right)`

`y+9=(-5)/(4)\left(x-8\right)`

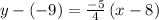
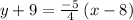
Writing the equation in slope-intercept form

`y=mx+b`

where m is the slope, and b is the y-intercept

so the equation of the line in slope-intercept form becomes

`y+9=(-5)/(4)\left(x-8\right)`

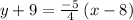
subtract 9 from both sides

`y+9-9=(-5)/(4)\left(x-8\right)-9`

`y=-(5)/(4)x+1`

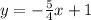
Therefore, the equation in the slope-intercept form will be:

`y=-(5)/(4)x+1`