Question

Allyson and Oliver have researched destinations and costs for a class trip. The Great Adventure theme park's entry fee is $15 plus they charge $1.50 at each ride using a Fast Pass. The Big Thicket amusement park charges $25 for its entry fee and $1.00 for each ride using a Fast Pass. To be able to ride all the rides, each student will need a Fast Pass for the day.

How many ride tickets must be purchased with the Fast Pass in order for the total cost at The Great Adventure theme park and The Big Thicket amusement park to be the same?
Record your answer and fill in the bubbles on your answer document. Be sure to use the correct place value.

Answer 1

20 tickets each will have to bought with fast pass so that the total cost is equal to that of each other.

Explanation:

Entry fee for The Great Adventure theme park = $15

Charge for fast pass at each ride = $1.50

Entry fee for The Big Thicket amusement park = $15

Charge for fast pass at each ride = $1.00

Let the number of ride tickets purchased with Fast pass =
`x`

As per question statement, the total cost at each will be equal to that of each other.

Writing in the equation form, we get:

$15 + $1.50
`*`
`x` = $25 + $1.00
`*`
`x`

`\Rightarrow 1.50x -1.00x = 25 -15\\\Rightarrow 0.50x = 10\\\Rightarrow x = 20`

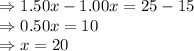
Therefore, 20 tickets each will have to bought with fast pass so that the total cost is equal to that of each other.