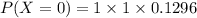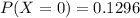Question

2. The probability that a car will come to a complete stop at a particular stop sign is 0.4. Assume that the next four cars

arrive at the stop sign are independent of one another. Find each of the following probabilities:
a) None of the four cars come to a complete stop?

Answer 1

`P(X = 0) = 0.1296`

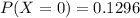
Explanation:

This can be solved using the binomial distribution because the cars arriving at the stop are independent of each other. This means we will use:

`P(X = x) = \binom{n}{x} p^(x) q^(n - x)`

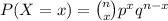
Where p is the probability of success, q = 1 - p is the probability of failure and n is the number of cars sampled.

p = 0.4

q = 0.6

n = 4

Therefore,

`P(X = 0) = \binom{4}{0} 0.4^(0) 0.6^(4)`

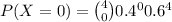
This is equal to

`P(X = 0) = 1 * 1 * 0.1296`

`P(X = 0) = 0.1296`