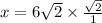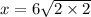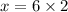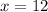Question

(someone please answer these. im literally not turning in my test until someone does. ) 1 a ladder is leaning agisnt a building so that the top of the ladder is touching the roof line. the bottom of the ladder is 8 feet from thr building and thr ladder is 17 feet long. how far is the roof line from the ground 15 ft, 9ft 18.8 ft 25ft

2 what is the value of x ?

Answer 1

1. 15ft

2. 12

Explanation:

1. See the attachment below showing the right triangle formed by the ladder and the building.

Let x represent how far the roof line is from the ground.

Thus, using Pythagorean Theorem, x can be solved as follows:

x² = 17² - 8²

x² = 225

x = √225

x = 15ft

2. Given:

Reference angle = 45°

x = Hyp

6√2 = opposite

Using trigonometric ratios formula, thus:

`sin(45) = (6√(2))/(x)`

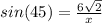
Multiply both sides by x

`sin(45)*x = 6√(2)`

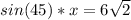
Divide both sides by sin(45)

`x = (6√(2))/(sin(45))`

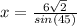
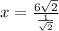`x = (6√(2))/((1)/(√(2)))` (common value of sin(45) = 1/√2)

`x = 6√(2) * (√(2))/(1)`

`x = 6√(2 * 2)`

`x = 6 * 2`

`x = 12`