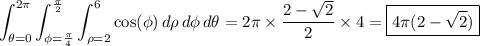Question

Please help me solve this problem.

Answer 1

Luckily, the integral is basically set up for you:

`\displaystyle \int_(\theta=0)^(2\pi) \int_(\phi=\frac\pi4)^(\frac\pi2) \int_(\rho=2)^6 \cos(\phi) \, d\rho \, d\phi \, d\theta`

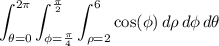
Since the limits on every variable are constant, and we can factorize
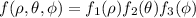`f(\rho,\theta,\phi) = f_1(\rho) f_2(\theta) f_3(\phi)`, we can similarly factorize the integrals. (This is a special case of Fubini's theorem, if I'm not mistaken.)

So the triple integral is equivalent to

`\displaystyle \left(\int_0^(2\pi) d\theta\right) \left(\int_(\frac\pi4)^(\frac\pi2) \cos(\phi) \, d\phi\right) \left(\int_2^6 d\rho\right)`

and each of these subsequent integrals are easy to compute:

`\displaystyle \int_0^(2\pi) d\theta = \theta \bigg|_0^(2\pi) = 2\pi - 0 = 2\pi`

`\displaystyle \int_(\frac\pi4)^(\frac\pi2) \cos(\phi) \, d\phi = \sin(\phi) \bigg|_(\frac\pi4)^(\frac\pi2) = \sin\left(\frac\pi2\right) - \sin\left(\frac\pi4\right) = 1 - \frac1{\sqrt2} = \frac{2 - \sqrt2}2`

`\displaystyle \int_2^6 d\rho = \rho\bigg|_2^6 = 6 - 2 = 4`

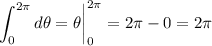
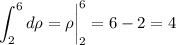
Taken together, the triple integral evaluates to

`\displaystyle \int_(\theta=0)^(2\pi) \int_(\phi=\frac\pi4)^(\frac\pi2) \int_(\rho=2)^6 \cos(\phi) \, d\rho \, d\phi \, d\theta = 2\pi * \frac{2-\sqrt2}2 * 4 = \boxed{4\pi(2-\sqrt2)}`