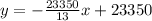Answer:
a. y-intercept = 23350 and x-intercept = 13
b.

c.
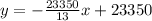
Explanation:
Given
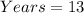
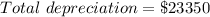
Solving (a): The x and y intercepts
The y intercept is the initial depreciation value
i.e. when x = 0
This value is the value of the car when it was initially purchased.
Hence, the y-intercept = 23350
The x intercept is the year it takes to finish depreciating
i.e. when y = 0
From the question, we understand that it takes 13 years for the car to totally get depreciated.
Hence, the x-intercept = 13
Solving (b): The slope
The slope (m) is the rate of depreciation per year
This is calculated by dividing the total depreciation by the duration.
So:
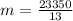
Because it is depreciation, it means the slope represents a deduction.
So,

Solving (c): The straight line equation
The general format of an equation is:

Where
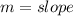

In (a), we have that:
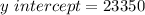
In (b), we have that:
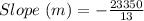
Substitute these values in

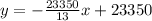
Hence, the depreciation equation is: