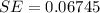Answer:
The mean is
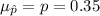
The standard error is
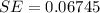
Explanation:
From the question we are told that
The sample size is n = 50
The population proportion is
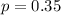
Generally given that the sample size is large enough , then the mean of the distribution is mathematically represented as
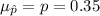
Generally the standard error of the distribution is mathematically represented as
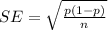
=>
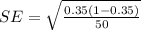
=>