Question

State whether the given measurements determine zero, one, or two triangles. b=86, b=21, c=18

Answer 1

Answer: 1

Explanation:

I assume you meant to type
`B=86^(\circ), b=21, c=18`.

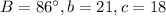By the Law of Sines,

`(\sin C)/(c)=(\sin B)/(b) \\ \\ (\sin C)/(18)=(\sin 86^(\circ))/(21) \\ \\ \sin C=(18 \sin 86^(\circ))/(21) \\ \\ C=\sin^(-1) \left((18 \sin 86^(\circ))/(21) \right), 180^(\circ)-\sin^(-1) \left((18 \sin 86^(\circ))/(21) \right) \\ \\ C \approx 58.77^(\circ), 121.23^(\circ)`

Since only one of these values will make a triangle (the obtuse possibility for C will mean
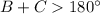`B+C > 180^(\circ)`, which violates the condition that the angles of a triangle add to 180 degrees), 1 triangle can be formed.