Question

The critical resolved shear stress for a metal is 35 MPa. Determine the maximum possible yield strength (in MPa) for a single crystal of this metal that is pulled in tension.

Answer 1

The maximum possible yield strength for a single crystal is 70 MPa.

Step-by-step explanation:

To find the maximum possible yield strength for a single crystal we need to use the following equation:

`\sigma_(y) = (\tau_(CRSS))/(m_(max))`

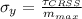
Where:

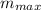`m_(max)` is the maximum value for Schmid factor

`\tau_(CRSS)` is the critical resolved shear stress = 35 MPa

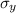`\sigma_(y)` is the yield strength =?

The Schmid factor is given by:

`m = cos(\phi)cos(\lambda)`

And its maximum value is obtained when λ = 45° and Φ = 45°, so:

`m = cos(45)cos(45) = 0.5`

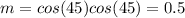
Finally, the maximum possible yield strength is:

`\sigma_(y) = (\tau_(CRSS))/(m_(max)) = (35 MPa)/(0.5) = 70 MPa`

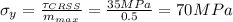
Therefore, the maximum possible yield strength for a single crystal is 70 MPa.

I hope it helps you!