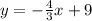Question

The position of an open-water swimmer is shown in the graph. The shortest route to the shoreline is one that is perpendicular to the shoreline. Write an

equation that represents this path.

Answer 1

`y = -(4)/(3)x + 9`

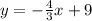
Step-by-sep explanation:

To create an equation that represents the shortest oath of the swimmer that is perpendicular to the shoreline, we need to find the slope, m, and the y-intercept, b.

The slope of the path would be the negative reciprocal of the slope of the shoreline, since they are perpendicular.

Let's find the slope of the shoreline.

Using two points on the shoreline, (0, 1) and (4, 4),

Slope (m) =
`(y_2 - y_1)/(x_2 - x_1) = (4 - 1)/(4 - 0) = (3)/(4)`

Therefore the slope, m, of the swimmer's path that is perpendicular to the shoreline would be -⁴/3.

Use the point of the swimmer and the slope of the path to find b.

Substitute m = -⁴/3, (x, y) = (6, 1) in
`y = mx + b`

`1 = (-(4)/(3))(6) + b`

`1 = -8 + b`

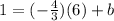
Add 8 to both sides

`1 + 8 = b`

`9 = b`

`b = 9`

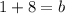
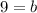
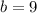
Now that we know the slope, m = -⁴/3, and the y-intercept, b = 9, plug in their values into
`y = mx + b`.

The equation of the path would be:

`y = -(4)/(3)x + 9`