2 Answers

Mob · Answer 1 · 2021-07-29T05:43:06+0000

Answer:

The correct option is;

a- 45° only

Explanation:

The given equation can be presented as follows;

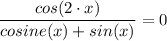
$(cos (2 \cdot x))/(cosine (x) + sin (x)) = 0$

We have that cos(2·x) = cos²(x) - sin²(x)

Also, we have, by the difference of two squares, the following relation;

cos²(x) - sin²(x) = (cos(x) - sin(x))(cos(x) + sin(x))

Therefore, the given equation can be written as follows;

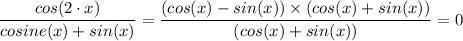
$(cos (2 \cdot x))/(cosine (x) + sin (x)) = ((cos(x) - sin(x)) * (cos(x) + sin(x)))/((cos (x) + sin (x))) =0$

Crossing the common term, (cos(x) + sin(x)), in the numerator and the denominator, we have;

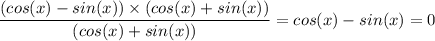
((cos(x) - sin(x)) * (cos(x) + sin(x)))/((cos (x) + sin (x))) = cos(x) - sin(x) = 0

From cos(x) - sin(x) = 0, we have;

Adding sin(x) to both sides of the equation

cos(x) - sin(x) + sin(x) = 0 + sin(x)

cos(x) = sin(x)

Therefore, the opposite leg and the adjacent leg of the right triangle formed with reference to the angle x are equal

∴ x = 90/2 = 45° only for 0° ≤ x ≤ 180°

Please log in or register to add a comment.