Function s is the set of points: {(4,3),(5,3),(8,6),(9,6),(12,9),(13,9)} (a) What is the inverse of function s?(b) the inverse of function s a function? Explain your answer.

Question

asked Jan 5, 2021 172k views

1 Answer

Mbcrump · Answer 1 · 2021-01-12T10:47:57+0000

Given:

The function in the form of set of points is

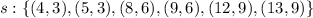
$s:\{(4,3),(5,3),(8,6),(9,6),(12,9),(13,9)\}$

To find:

(a) The inverse of function s.

(b) whether the inverse of function s is a function?

Solution:

(a)

If a function is
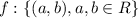$f:\{(a,b),a,b\in R\}$ , then its inverse function is

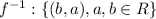
$f^(-1):\{(b,a),a,b\in R\}$

We have,

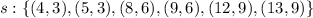
$s:\{(4,3),(5,3),(8,6),(9,6),(12,9),(13,9)\}$

So, inverse of this function is

$s^(-1):\{(3,4),(3,5),(6,8),(6,9),(9,12),(9,13)\}$

Therefore, the inverse of function s is
$s^(-1):\{(3,4),(3,5),(6,8),(6,9),(9,12),(9,13)\}$ .

(b)

A set of point is a function if there exist unique output for each input.

In inverse of s, we have two output values y=4 and y=5 for single input x=3.

Therefore, the inverse of function s is not a function.