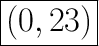
Hey there! We'll solve this problem in three main steps. First, we'll find the slope of the line. Then, we'll find the equation line in point-slope form. Finally, we'll change the equation to slope-intercept form so we can tell what the y-intercept is.
We can use the slope formula, where
 and
and
 are two known points on the line.
are two known points on the line.
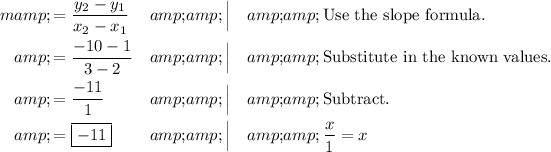
Now that we have the slope, we can use it together with one of the points to get the line in point-slope form. Then, we can distribute and add to get the line in slope-intercept form.

We can now clearly see that the y-intercept of the line is
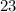 , or
, or
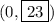 as a point.
as a point.